This set of Class 11 Physics Chapter 14 Multiple Choice Questions & Answers (MCQs) focuses on “Oscillations – Energy in Simple Harmonic Motion”.
1. What is the time period of kinetic energy of a particle in SHM,if the time period of SHM is 4s?
a) 2s
b) 1s
c) 4s
d) 0s
View Answer
Explanation: The kinetic energy of a particle in SHM is given by:
0.5mA2w2cos2(wt + a), where a is the phase constant.
cos2(wt + a) = (1 + cos2(wt+a))/2.
Therefore the time period of oscillation of kinetic energy is 4/2 = 2s.
2. Consider a particle undergoing an SHM of amplitude A & angular frequency w. What is the magnitude of displacement from the mean position when kinetic energy is equal to the magnitude of potential energy?
a) A/2
b) A/√2
c) A/3
d) A/4
View Answer
Explanation: Let x = Asin(wt).
∴ Kinetic energy = 1/2 mA2w2cos2(wt)
potential energy = 1/2kA2sin2(wt).
According to given condition:
1/2mA2w2cos2(wt) = 1/2A2w2sin2(wt)
using, k = mw2, we get:
tan2(wt) = 1.
∴ wt = π/4.
∴ x = Asin(π/4) = A/√2.
3. The graph given below represents energy as a function of position. What is the energy of the particle in SHM at x = +1? Given mass of the particle is 2kg, time period =2s, A=1m.
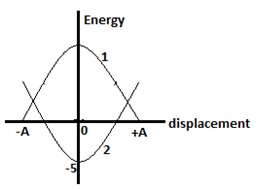
a) 4.87J
b) -5J
c) 9.87J
d) 5J
View Answer
Explanation: w = 2π/T = π.
Maximum kinetic energy = 1/2mA2w2, which will be at x=0.
Total energy will be constant,
therefore energy at x=0 is the same as the energy at x = +1.
Thus, energy = 1/2mA2w2 – 5
= (0.5*2*1*9.87) – 5
= 4.87J.
4. A particle is undergoing SHM. If its potential energy is given by: U = kx2. What will be the value of x for potential energy to be 1/3rd of kinetic energy? Assume k is force constant.
a) A/√7
b) A/√6
c) A/√3
d) A/√2
View Answer
Explanation: Let x = Asin(wt).
U = kA2sin2(wt)
K = 1/2mA2w2cos2(wt).
Given: U = 1/3K.
kA2sin2(wt) = (1/3)*(1/2)mA2w2cos2(wt),
and k = mw2.
∴ tan2(wt) = 1/6. Or tan(wt) = 1/√6.
∴ sin(wt) = 1/√7.
x = Asin(wt) = A/√7.
5. A particle is performing a SHM. If its mass is doubled keeping the amplitude and force constant the same, total energy will become how many times the initial value?
a) 2
b) 1/2
c) 4
d) 1
View Answer
Explanation: Total energy = 1/2mw2A2.
So, if mass is doubled keeping the amplitude same,
total energy will get doubled.
6. A particle is in SHM. When displacement is ‘a‘ potential energy is 8J. When displacement is ‘a‘ potential energy is 18J. What will be the potential energy when displacement is ‘a+b’?
a) 50J
b) 26J
c) 10J
d) 30J
View Answer
Explanation: 1/2ka2 = 8 & 1/2kb2 = 18.
We have to find value of 1/2k(a + b) 2.
∴ 1/2k(a + b)2 = 8 + 18 + kab
= 26+(k*4*6/k)
= 26 + 24 = 50J.
7. If the total energy of a particle in SHM is 200J, the value of kinetic energy at an instant can be 220J. True or False?
a) True
b) False
View Answer
Explanation: The total energy of the particle is the sum of kinetic & potential energy. Potential energy is frame dependent, so it can be negative in some cases. Thus, the value of kinetic energy can exceed total energy.
8. If the potential energy of the particle is given by: U = x2-8x+16. It starts from rest from x=0. What will be its maximum speed during SHM? Assume the mass of the particle to be 1kg.
a) 4√2m/s
b) 8m/s
c) 2√2m/s
d) 2√6m/s
View Answer
Explanation: F = -dU/dx = 8 – 2x.
Particle starts from x=0, so force will increase its speed till force becomes zero at x=4.
Let acceleration be ‘a’.
a = dv/dt = v dv/dx.
And a = F/m = F/1 = 8-2x.
adx = vdv. Now, integrate both sides. The limits of dx will be 0 to 4 and the limits of dv will be 0 to v.
(8x – x2)04 = v2/2.
∴ v = 4√2 m/s.
Sanfoundry Global Education & Learning Series – Physics – Class 11.
To practice all chapters and topics of class 11 Physics, here is complete set of 1000+ Multiple Choice Questions and Answers.
If you find a mistake in question / option / answer, kindly take a screenshot and email to [email protected]
- Practice Class 12 - Physics MCQs
- Check Class 11 - Physics Books
- Practice Class 11 - Mathematics MCQs
- Practice Class 11 - Chemistry MCQs
- Practice Class 11 - Biology MCQs
