This set of Class 11 Physics Chapter 7 Multiple Choice Questions & Answers (MCQs) focuses on “Angular Momentum in case of Rotations about a Fixed Axis”.
1. A particle is rotating about a fixed axis. The angular momentum of the particle about any point on the fixed axis is the same. True or False?
a) True
b) False
View Answer
Explanation: The angular momentum of a particle rotating about a fixed axis is given by L = r X p. At any time the vector p is fixed. But from different points on the axis, we will get different r vectors. So, the cross product of r & p will be different. Hence, the angular momentum vector is different about different points on the axis.
2. The angular momentum vector of a particle about a point on the rotational axis is parallel to its angular velocity vector, provided the particle rotates with fixed speed about that axis. True or False?
a) True
b) False
View Answer
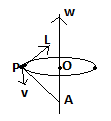
Explanation: The diagram below shows that the angular momentum of the particle is not along the direction of angular velocity when calculated about point A. Both the vectors are parallel when the angular momentum is calculated about point O.
3. What is the rate of change of angular momentum?
a) Force
b) Torque
c) Work
d) Angular velocity
View Answer
Explanation: Let L, r & p be the angular momentum, radius & momentum vectors respectively. Angular momentum of a body is ∑(r X p). On differentiating w.r.t time, dL/dt = ∑{dr/dt X p + r X dp/dt} = ∑{v X mv + r X F} = ∑{r X F} = total torque ‘τ’.
Here, F is the total force vector. And v X mv is zero because the cross product of two vectors in the same direction is zero.
4. Which of the following quantities is zero if torque on a particle is zero?
a) Angular momentum of the particle
b) Angular speed of the particle
c) Change in kinetic energy of the particle
d) Rate of change of angular momentum
View Answer
Explanation: Torque is defined as the rate of change of angular momentum. So, if torque is zero, the rate of change of angular momentum will be zero. An important thing to note is that one may think how kinetic energy can change if torque is zero. For this consider the example of a ballet dancer, when she/he pulls in her hands while rotating she/he becomes faster but angular momentum remains constant since net torque is zero. But her kinetic energy = 1/2 (I) will increase as has increased.
5. A particle of mass 5kg is rotating about an axis in a circle with a speed of 10m/s. What should be its radius so that the component of angular momentum about the axis is 5kgm2/s?
a) 0.1cm
b) 10cm
c) 5cm
d) 0.5m
View Answer
Explanation: The angular momentum of a particle along the axis is given by Iω. I = mr2 ω = v/r, where ‘r’ is the radius of the particle. Iω = mvr = 5 ∴ 5*10*r = 5 ∴ r = 0.1m = 10cm.
6. A disc is rotating with ω =10rad/s about a fixed central axis which is perpendicular to its plane. The disc has a mass =2kg & radius =10cm. A small particle of mass 100gm is put slowly on the disc’s outer circumference. There is sufficient friction between the disc and the particle. What will be the new angular velocity of the system?
a) 10 rad/s
b) 9.09 rad/s
c) 9.51 rad/s
d) Can’t conserve angular momentum because of friction
View Answer
Explanation: The friction acting between the two surfaces will ensure that the particle stays on the disc and doesn’t fly out. The angular momentum of the system will be conserved because there is no external torque. Moment of inertia of disc = MR2/2 = 2*0.01/2 = 0.01kgm2.
Moment of inertia with particle on disc = 0.01 + mR2
= 0.01+0.1*0.01 = 0.011kgm2.
∴ I1w1 = I2w2
∴ 0.01*10 = 0.011*w2
∴ w2 = 9.09 rad/s.
7. A hollow rod has frictionless inner walls. Inside the rod are two small spheres that are kept on either side of the centre of rod. The rod is rotated about its central axis which is perpendicular to the plane of rotation. If the rod had been rotated by an impulsive torque which gave it an instantaneous angular velocity of 5rad/s, what will be the angular velocity after some finite but long time ‘t’? Assume no external forces act on rod after the impulse. Also state what will happen to the spheres in the centre of the rod. The rod has a mass =2kg & length =10cm. The two spheres have a mass =1kg each.
a) 2.5 rad/s, spheres will stay where they are as there is no friction to move them
b) 0 rad/s, spheres will move outwards and decrease velocity of rod to zero
c) 1.25 rad/s, spheres will move to opposite ends of rod
d) 2.5 rad/s, spheres will move to opposite ends of rod
View Answer
Explanation: When the rod gets an impulse, it gets an initial angular velocity. Now, the two spheres will start going outwards till they reach the end of the rod on either side as the walls of rod’s ends will provide the required centripetal force. Also, to find final angular velocity we can conserve angular momentum as there is no external torque.
I1w1 = I2w2,
where I1, I2, w1, w2 are the initial & final moment of inertias & angular velocities resp.
I1 = MI2/12 + 0 = 2*0.01/12 = 0.01/6 kgm2.
I1 = MI2/12 + 2*mI2/4
= (0.01/6) + (0.5*1*0.01)
= (0.01/6) + (0.01/2)
= 0.04/6 = 0.02/3 kgm2.
∴ I1w1 = I2w2 0.01/6 * 5 = 0.02/3 *w2
∴ w2 = 1.25 rad/s.
8. A ring and a disc having masses in the ratio 1:2 are made to rotate about their central axes. Both are acted upon by the same torque when they are at rest. Which one will have more angular velocity when torque has been removed from both after the same time? Both have the same radius.
a) Ring
b) Disc
c) Same for both
d) Depends on ratio of mass and radius
View Answer
Explanation: The angular momentum for each will be the same as torque is the same. Let the mass of the ring be m, and their radius be ‘R’. The ratio of angular speeds will be the inverse ratio of their moments of inertia. Moment of inertia of ring = mR2& moment of inertia of disc = 2mR2/2 = mR2. As their moment of inertia is the same their angular speeds will be the same.
Sanfoundry Global Education & Learning Series – Physics – Class 11.
To practice all chapters and topics of class 11 Physics, here is complete set of 1000+ Multiple Choice Questions and Answers.
If you find a mistake in question / option / answer, kindly take a screenshot and email to [email protected]
- Practice Class 11 - Chemistry MCQs
- Practice Class 12 - Physics MCQs
- Check Class 11 - Physics Books
- Practice Class 11 - Biology MCQs
- Practice Class 11 - Mathematics MCQs
