This set of Computational Fluid Dynamics Multiple Choice Questions & Answers (MCQs) focuses on “Mathematical Behaviour of PDE – Hyperbolic Equations”.
1. Consider the following diagram.
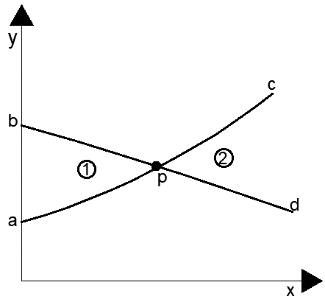
What does line ‘ab’ represent?
a) Left characteristic line
b) Right characteristic line
c) Data which depends on P
d) Initial data on which P is dependent
View Answer
Explanation: Line ‘ab’ is the initial data which P depends on. They influence the values of dependent variables at point P. Solutions march from this line.
2. When the hyperbolic equations are represented by a diagram, what is the region dependent on the point of consideration called?
a) Boundary region
b) Domain of dependence
c) Region of influence
d) Initial region
View Answer
Explanation: The area which is affected by point P is called the region of influence. Any disturbance at that point will disturb the solution in the region of influence.
3. Consider the diagram.

In the diagram what does region 1 mean?
a) Region of influence
b) Domain of dependence
c) Characteristic region
d) Boundary region
View Answer
Explanation: The values at point P depend upon the region ahead of P. So, it is called the domain of dependence. This starts from the line ‘ab’.
4. When is the steady inviscid flow hyperbolic?
a) In supersonic flow regime
b) Never
c) Always
d) In subsonic flow regime
View Answer
Explanation: In subsonic speeds, steady inviscid flow is elliptic. When the Mach number is more than 1, they become hyperbolic.
5. Which of these is true for hyperbolic equations?
a) They have 2 imaginary characteristic lines
b) They have 1 imaginary characteristic line
c) They have 1 real characteristic line
d) They have 2 real characteristic lines
View Answer
Explanation: Hyperbolic equations get a positive determinant. Taking the square root of this positive determinant, we will get two real characteristic lines.
6. From the diagram,

What does, line Pc represent?
a) Left running boundary line
b) Right running characteristic line
c) Left running characteristic line
d) Left running boundary line
View Answer
Explanation: Lines ‘ac’ and ‘bd’ represent the characteristic lines of the hyperbolic problem. From point P if we face the direction of marching, the left and right side characteristic lines are called the left and right running characteristic lines.
7. Which of these flows can be represented by the following diagram?

a) Unsteady viscous supersonic flow
b) Unsteady inviscid supersonic flow
c) Steady inviscid supersonic flow
d) Steady inviscid subsonic flow
View Answer
Explanation: From the diagram, as the solutions are time marching, the flow must be unsteady. Unsteady viscous flow is parabolic. But the diagram is for hyperbolic problems. So, unsteady inviscid supersonic flow suits this diagram.
8. To solve a hyperbolic equation, which of these is/are necessary?
a) Initial conditions and boundary conditions
b) Initial conditions
c) Boundary conditions
d) Neither initial nor boundary conditions
View Answer
Explanation: For solving any hyperbolic problem, initial conditions are needed to march from and boundary conditions are needed additionally.
9. Which of these equations is hyperbolic?
a) Unsteady Navier-Stokes equation
b) Steady Navier-Stokes equation
c) Steady Euler equation
d) Unsteady Euler equation
View Answer
Explanation: Euler equations represent inviscid flows. Unsteady inviscid flows are always hyperbolic. Steady inviscid flows are conditionally hyperbolic.
10. The speed of propagation of the disturbance in hyperbolic problems is ____________
a) finite
b) infinite
c) 0
d) 1
View Answer
Explanation: Disturbances propagate at a finite speed defined by some flow properties. In the elliptic and parabolic equations, this speed is infinite.
Sanfoundry Global Education & Learning Series – Computational Fluid Dynamics.
To practice all areas of Computational Fluid Dynamics, here is complete set of 1000+ Multiple Choice Questions and Answers.
If you find a mistake in question / option / answer, kindly take a screenshot and email to [email protected]
- Apply for Mechanical Engineering Internship
- Practice Chemical Engineering MCQs
- Check Chemical Engineering Books
- Practice Mechanical Engineering MCQs
- Apply for Chemical Engineering Internship
