This set of Structural Analysis Multiple Choice Questions & Answers (MCQs) focuses on “Moment-Area Theorems – 2”.
Point A is pin joint and B is roller. A load of 40KN is applied at point C.
Length of AC is 2m and d same goes for beam CB.
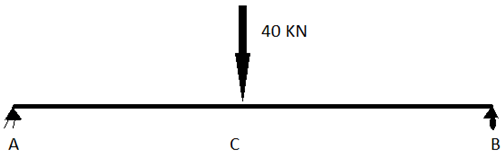
1. What is the shape for SFD of this diagram?
a) Linear
b) Parabolic
c) Linear with discontinuity
d) Arbitrary curve
View Answer
Explanation: Since loads are not uniform, SFD will be linear and loading at point C will lead to discontinuity.
2. What is the shape of BMD for this diagram?
a) Rectangular
b) Triangular
c) Parabolic
d) Arbitrary curve
View Answer
Explanation: It will increase till point C and then will start decreasing and in both cases linearly.
3. Where is the peak point of SFD?
a) A
b) C
c) B
d) between A and C
View Answer
Explanation: Value of shear force at point A is +20KN which is the maximum for this case.
4. What is the peak value of BMD of this figure (all options are in KN-m)?
a) 10/EI
b) 20/EI
c) 30/EI
d) 40/EI
View Answer
Explanation: At point A BM will be 0 and hen will start increasing till point C where its value will be 40/EI i.e. the area between SFD till that point.
5. What should be the area that should be considered if we want to find slope at point B wrt initial beam?
a) from A to C
b) from A to B
c) from C to B
d) depends upon the loading condition
View Answer
Explanation: If we thee deflected diagram of this figure, we will find that slope at point C will be zero as from that point, BM starts decreasing. So we should consider area between point C and B for slope wrt initial beam.
6. What is the value of slope at point B wrt initial beam?
a) 10/EI
b) 20/EI
c) 30/EI
d) 40/EI
View Answer
Explanation: Just calculate the area between point C and point B.
7. To calculate maximum deformation in the deflected beam, which part of the area should be considered?
a) from A to B
b) from A to C
c) from B to C
d) can’t say
View Answer
Explanation: Maximum deformation happens at point C and slope of C is zero i.e. tangent is parallel to initial beam. This property of point C can be used to calculate maximum deformation.
8. To calculate maximum deformation in deflected beam, about which point should we take moment of the required part of area?
a) A
b) B
c) C
d) can’t say
View Answer
Explanation: Tangent at point A is at point A as there is no vertical deflection there and tangent of point C is parallel to initial beam. So, the length at which it will cut point A vertically will be the maximum deformation.
9. What is the value of maximum deformation in this case?
a) 80/3EI
b) 120/3EI
c) 180/3EI
d) 40/3EI
View Answer
Explanation: Just take area between point A and C and calculate moment about point A.
10. To calculate relative deflections of tangents at point A and B at point B, about which point should we moment of the required part of area?
a) A
b) B
c) C
d) can’t say
View Answer
Explanation: This is the basic second theorem of moment-area theorem.
11. To calculate relative deflections of tangents at point A and B at point B, which part of area should be considered?
a) A to B
b) A to C
c) C to B
d) can’t say
View Answer
Explanation: This is the basic first theorem of the moment-area theorem.
12. What will be the value of relative deflections of tangents at point A and B at point B?
a) 20/3EI
b) 40/3EI
c) 80/3EI
d) 160/3EI
View Answer
Explanation: On doing the steps suggested in Q11 and Q10, we will get this result.
Sanfoundry Global Education & Learning Series – Structural Analysis.
To practice all areas of Structural Analysis, here is complete set of 1000+ Multiple Choice Questions and Answers.
If you find a mistake in question / option / answer, kindly take a screenshot and email to [email protected]
- Apply for Civil Engineering Internship
- Practice Civil Engineering MCQs
- Check Civil Engineering Books
- Check Structural Analysis Books
