This set of Structural Analysis Questions and Answers for Entrance exams focuses on ” Analysis of Beams-3″.
A and C are fixed supports. B is a roller. A distributed load is acting on beam BC with peak at C being 6N/ft. AB is 8 ft. while BC is 6ft. Take EI as constant.
All moments options are in N-ft.
1. What will be the value of mCB, after solving these equations?
a) 3.09
b) 1.54
c) 12.86
d) -3.09
View Answer
Explanation: Just substitute the value of rotation at point B in 4th equation.
2. What will be the value of mBA, after solving these equations?
a) 3.09
b) 1.54
c) 12.86
d) -3.09
View Answer
Explanation: It will be inverse of mBC as shown above.
3. What will be the shear at point A?
a) -0.379
b) 0.479
c) -0.579
d) 0.679
View Answer
Explanation: Just conserve moment in beam AB about point B.
4. What will be the shear at point C?
a) -11.86
b) 12.86
c) -13.86
d) 14.86
View Answer
Explanation: Just conserve moment in beam BC about point B.
5. What will be the support reaction at point B?
a) 4.95
b) -5.95
c) 3.65
d) can’t say
View Answer
Explanation: Find shear at point B in both beam AB and BC and then cut a small part near support B and conserve force in vertical direction.
A is a fixed support, while B and C are roller supports. Uniformly distributed load of 2KN/m is acting on span AB. Load of 12 kN acts at a point between B and C. AB = 24m, BC = 8m. Load of 24KN acts at centre of BC.
All moment options are given in kN-M.
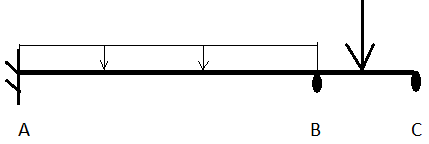
6. How many separate parts will be required for this question?
a) 0
b) 1
c) 2
d) 3
View Answer
Explanation: Since, 3 supports are there we will divide it into 2 separate parts to solve.
7. What is FEM of point A in beam AB?
a) 18
b) -18
c) 96
d) -96
View Answer
Explanation: Formula for moment for unit load applied at mid point is WL2.12 and direction will be anti clockwise.
8. What is FEM of point C in beam BC if we assume beam BC to be fixed?
a) 12
b) -12
c) 36
d) -36
View Answer
Explanation: Formula for moment for unit load applied at mid point is PL/8 and direction will be clockwise at point C.
9. What will be the end moment at point B in beam BC?
a) 18
b) -18
c) 96
d) -96
View Answer
Explanation: Formula for moment for unit load applied at mid point is WL^2.12 and direction will be clockwise.
10. What will be the end moments at point B in beam BC if beam BC is considered to be fixed at support B and pin supported at support C?
a) 18
b) -18
c) 96
d) -96
View Answer
Explanation: In that case, moment at C will be zero and half of that moment will be transferred to point B.
11. What will be the deflection of beam AB?
a) 0
b) 1/EI
c) 2/EI
d) can’t say
View Answer
Explanation: As, point A is a fixed support, it won’t allow any deflection to take place.
12. What will be the deflection of beam BC?
a) 0
b) 1/EI
c) 2/EI
d) can’t say
View Answer
Explanation: As, point B is a fixed support, it won’t allow any deflection to take place.
13. What will be the rotation of beam AB at point A?
a) 0.2
b) -0.2
c) 0
d) can’t say
View Answer
Explanation: There won’t be any rotation at point A as it is a fixed support.
14. What will be the rotation of beam BC at point C?
a) 0.2
b) -0.2
c) 0
d) it will depend upon the rotation of beam BC at point B
View Answer
Explanation: According to equations, it will depend upon the rotation of beam BC at point C.
15. After using all the joint conditions, how many unknowns are still left?
a) 0
b) 1
c) 2
d) 3
View Answer
Explanation: Rotation at point B in either of beam is not known (they are both equal).
Sanfoundry Global Education & Learning Series – Structural Analysis.
To practice all areas of Structural Analysis for Entrance exams, here is complete set of 1000+ Multiple Choice Questions and Answers.
If you find a mistake in question / option / answer, kindly take a screenshot and email to [email protected]
- Check Structural Analysis Books
- Apply for Civil Engineering Internship
- Practice Civil Engineering MCQs
- Check Civil Engineering Books

