This set of Control Systems Questions and Answers for Experienced people focuses on “Time Response of Second Order Systems – III”.
1. The unit impulse response of a second order system is 1/6e-0.8tsin(0.6t). Then the natural frequency and damping ratio of the system are respectively.
a) 1 and 0.6
b) 1 and 0.8
c) 2 and 0.4
d) 2 and 0.3
View Answer
Explanation: 1/10[1/(s2+1.6s+1)] w = 1 rad/s
2Gw = 1.6
G = 0.8.
2. In a RLC series circuit, if the resistance R and inductance L are kept constant but capacitance C is decreased, then which one of the following statements is/are correct?
1. Time constant of the circuit is changed.
2. Damping ratio decreases.
3. Natural frequency increases.
4. Maximum overshoot is unaffected.
a) 1 and 2
b) 2 only
c) 2 and 3
d) 3 and 4
View Answer
Explanation: G = R/2[√C/L] C decreases, G decreases i.e., damping ratio decreases w = 1/√LC
Time constant = 2L/R
As C decreases, time constant remains unaffected and Natural frequency increases.
3. Consider the following system shown in the diagram :
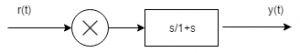
If the system shown in the above diagram x (t) = sint. What will be the response y (t) in the steady state?
a) sin(t-45)/√2
b) sin(t+45)/√2
c) √2e-5sint
d) sint-cost
View Answer
Explanation: Y(s) = [-1/2]/s+1+ [1/2]/(s^2+1) + [s/2]/(s^2+1)
y(t)= 1/2e-t+ ½[cost+sint].
4. For critically damped second order system, if the gain constant(K) is increased, the system behavior
a) Becomes oscillatory
b) Becomes under damped
c) Becomes over damped
d) Shows no change
View Answer
Explanation: Gain of the closed loop system is inversely proportional to the damping and hence if the gain of the system is increased then the damping is reduced and less than 1 system becomes under damped.
5. As unity feedback system has a forward path transfer function G(s) = K/s(s+8) where K is the gain of the system. The value of K, for making this system critically damped should be
a) 4
b) 8
c) 16
d) 32
View Answer
Explanation: Overall transfer function M(s) = K/K+s(s+8)
Therefore the characteristic equation is s2+8s+K
w = √K , 2Gw = 8
w = 4 and K = 16.
6. Assertion (A): It is desirable that the transient response be sufficiently fast and sufficiently damped.
Reason (R): Oscillations are not tolerated in the transient response.
a) Both A and R are true but R is not the correct explanation of A
b) Both A and R are true but R is correct explanation of A
c) A is true and R is false
d) A is false and R is true
View Answer
Explanation: Oscillations must not be present in the transient response as it makes the response slow and sluggish and with positive feedback oscillations are increased and hence positive feedback is not used frequently.
7. The maximum overshoot and rise time conflict with each other.
a) True
b) False
View Answer
Explanation: For the system to be stable the rise time must be less so that the speed of response is increased and maximum peak overshoot should also be less.
8. The maximum overshoot is:
a) To measure the relative stability
b) A system with large overshoot is desirable
c) It occurs at second overshoot
d) Both b and c
View Answer
Explanation: To measure the relative stability ,the maximum overshoot is used. It is given a time domain specification and the system is desirable with small overshoot and it occurs at first overshoot.
9. The second order approximation using dominant pole concept of a system having transfer function 5/(s+5)(s2+s+1) is:
a) 5/(s2+s+1)
b) 1/(s2+s+1)
c) 1/(s+5)(s+1)
d) 5/(s+5)(s+1)
View Answer
Explanation: Given, T.F. = 5/(s+5)(s2+s+1)
Neglecting the insignificant pole, s=5
T.F = 1/(s2+s+1).
10. For the system, C(s)/R(s) = 16/(s2+8s+16). The nature of the response will be
a) Overdamped
b) Underdamped
c) Critically damped
d) None of the mentioned
View Answer
Explanation: Compare the equation with the characteristic equation s2+2Gws+w2. Then the value of w = 4 and value of G = 1 hence the system is critically damped with poles on the imaginary axis.
Sanfoundry Global Education & Learning Series – Control Systems.
To practice all areas of Control Systems for Experienced people, here is complete set of 1000+ Multiple Choice Questions and Answers.
If you find a mistake in question / option / answer, kindly take a screenshot and email to [email protected]
